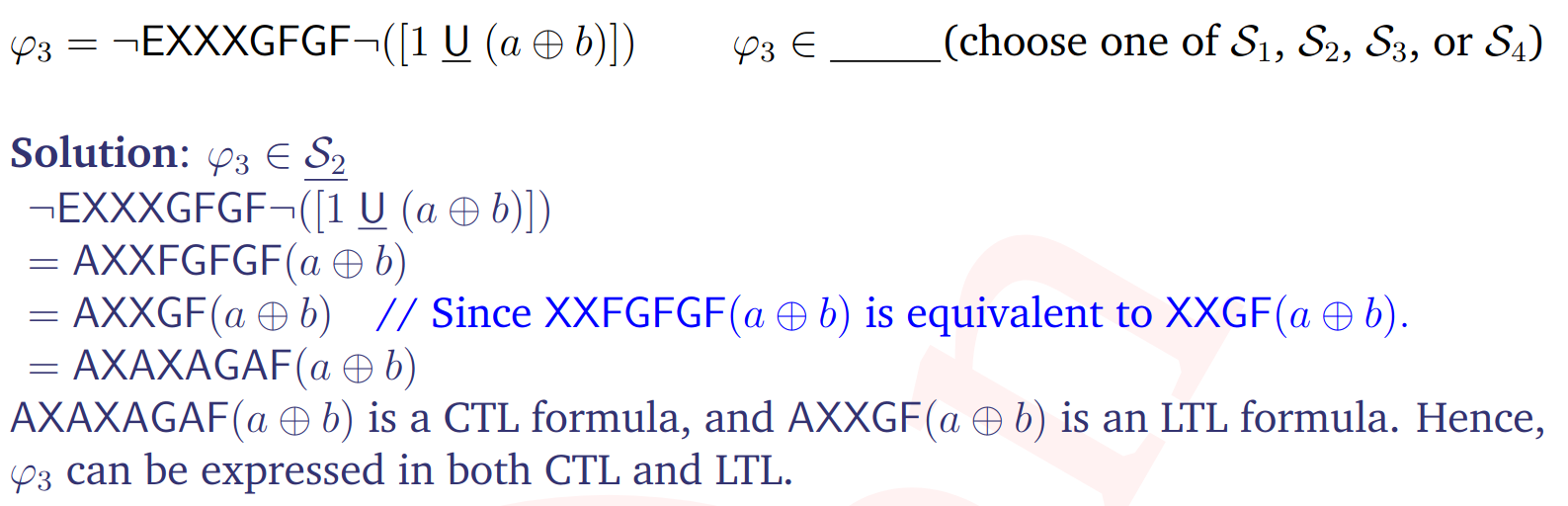
Hello! In the above problem (from 14.02.2018) for translation of the formula, I have a different solution to the one given in the image above.
Is it accepted answer if I translate with the following steps:
= AXXFGFGF(a ⊕ b)
= AXXGF(a ⊕ b) (because: Alternation of F,G ending with F = GF)
= AGF(a ⊕ b) (because: XXGF = GF)
= AGAF(a ⊕ b)
Note: I came across the rule XXGF = GF from the solutions stated in the paper dated 27.08.2021, an image of which is attached below:
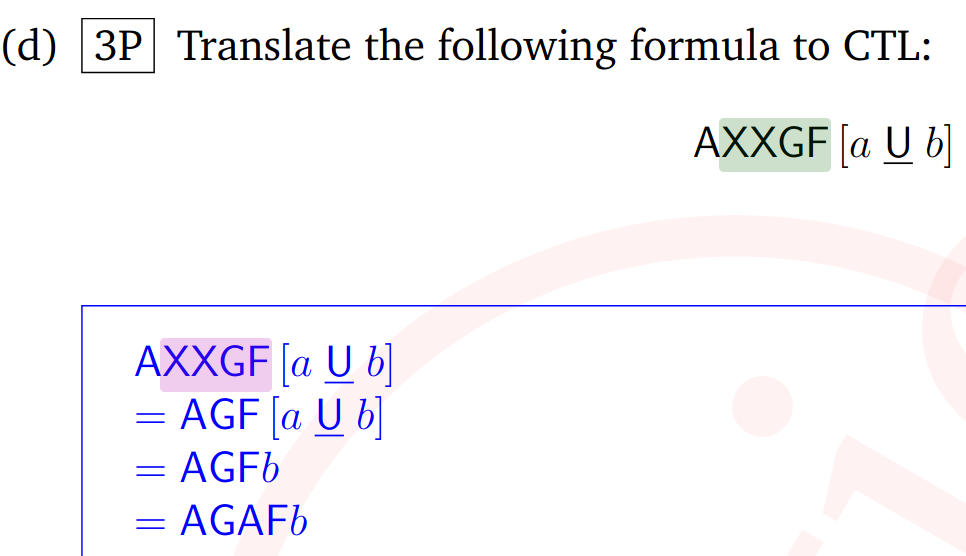
Thanks for your support.!